Measuring the dimensions of your dam
Your dam may not be as deep as you think! After a few storms and flooding, and years of livestock and loose dry pasture, most dams accumulate a lot of silt and organic material in the base. Desilting dams and building silt traps may be a worthwhile investment.
With a few tools and some preparation, the method below gives a useful estimate of dam volume. It can be difficult to measure the dimensions of dams in use (unless empty), and calculated volumes are approximate. For water budgeting, always assume the usable volume is 10% less than the calculated volume, and allow for evaporation and difficulty of pumping or using the last 0.5 metre (m) of water in the dam.
At a minimum, you need to know the surface area of water in the dam and the maximum depth of water. For more accurate estimates of volume, you also need to measure the dam floor.
Simple, rough estimate of current water volume
Volume (V) in cubic metres (m3) = surface area in square metres (m2) x the maximum depth in metres (m) x 0.4
The 0.4 factor allows for the standard slope (3:1) of the internal walls of an excavated farm dam. For most farm dam sizes, this calculation will underestimate the water volme, but this provides some reserve.
For rectangular or square dams, pace (in metre steps) the length (L) and width (W) of the bank at the water surface. Surface area is L x W in square metres.
For example, for a dam with the water edge 60m long and 40m wide, and 5m depth:
V = 60 x 40 x 5 x 0.4 = 4800m3 = 4 800 000 litres- For round dams, pace the circumference (C) of the bank at water level. The equation to get the area (A) from a known circumference is: A = C2 / 4π, where π = 3.142 approximately.
For example, for a round dam with a circumference of 150m, and 6m depth:
V = 1502 x 6 x 0.4 / (4 x 3.142) = 4297m3 = 4 297 000 litres
Measuring the depth of water in a dam
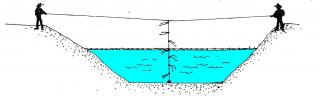
One way is to use a 'dam dipper' as in Figure 1. This needs 2 people to hold a string across the middle of the dam, with a weighted string attached at about the mid-point. The weighted string should be longer than the full depth of water, and have coloured markers tied to it at set distances so the depth can be seen from the side of the dam. Allow the weight at the end of the string to rest on the dam floor.
On large dams, it may be easier to launch a canoe and measure from the canoe.
Using a dam volume table to estimate water volume
The dam volume table in Figure 2 will help you to:
- estimate the dimensions of a dam when pegging a new site
- estimate the capacity of a completed dam
- calculate the volume of water in an existing dam
- estimate the remaining water in a dam after a given rate of water use.
The table refers to conventional excavated dams (earth tanks) of square plan on a level site, with 3:1 batter slopes. Most browsers allow you to right-click on the opened figure (click on it once to open it) and save the image to print out.
The capacity of a full dam
To estimate the volume capacity of a full, square dam, measure its depth – from the bottom of the dam to the overflow level – and the average length of the 4 top edges of the excavation at overflow level (see the body of the table in Figure 2). At the measured depth, scan down the column to the nearest top edge dimension. The volume can then be read from the left-hand column of the table.
Calculating the water volume left in a dam
To estimate the volume of water in an existing square dam, measure the depth of water (see the technique above) and the length of 1 side of the water surface (for a square dam). Example 1 below shows how to use your measurments to estimate the dam volume.
Example 1: volume calculation from the table in Figure 2
Depth = 5.25m (top line in table)
Length of 4 top sides of excavation = 46.4m, 47.2m, 46.1m, 47.5m
Average length = (46.4 + 47.2 + 46.1 + 47.5)/4 = 187.2/4 = 46.8m (from the column below the depth reading)
Volume = 5500m3 (reading from the left-hand column)
Note that the consumption curve '15' intersects 46.8 on the 5.25m column.
Consumption curves in Figure 2
Assuming that leakage is minimal, water will be lost from the dam from evaporation and from water extracted for livestock or other uses.
The curves in Figure 2 show how the water volume decreases with decreases in water depth.
Use monthly consumption steps equal to evaporation (in metres along the top) and livestock supply (in cubic metres up the side) to move along the curve.
The consumption curve can also be used to estimate leakage from the dam. See treating leaky farm dams for more information about leakage.
Allowing for evaporation
For the dam in example 1 above, if the evaporation for a summer month was 250mm (0.25m), move from the 5.25m depth column to the 5.00m depth column; move down the column to the consumption curve that intersects 46.8. The new side length is 45.4m and the new volume is shown to the left in that row, 5000m3.
Maps of evaporation are available on the Bureau of Meteorology website.
Allowing for livestock use
For the dam in example 1 above, if the dam is the water supply for 500 dry adult sheep in a summer month, the dam would need to supply about 10 litres (L) per head per day:
500 (sheep) x 10 (L/day) x 30 (days in the month) = 150 000L = 150m3
To find the new dam depth, move from the 5000m3 volume to 4850m3 (roughly near the 4750m3 row); move along that row to where the row intersects the previously mentioned consumption curve, just to the left of the 44.6m side length entry; then move up that column to the depth value, which is now about 4.85m.
Combined evaporation and livestock water loss in 1 month was 5500m3 minus 4850m3 = 650m3, and the depth dropped from 5.25m to 4.85m.
See the livestock water requirements web page for more information.
Calculating farm dam leakage losses
Small leakages of about 2 millimetres (mm) off the depth per day are acceptable in farm dams, and would be hard to measure. Larger leakages can be estimated from the table in Figure 2 if you know the local evaporation rate and can accurately measure the water depth.
Losses of 10mm per day (300mm for the month) will give a result very much like the evaporation loss above. To measure that leakage, prevent livestock consumption for a month, measure evaporation loss, and use the evaporation example: evaporation of 250mm and leakage of 300mm would drop the dam volume from 5500m3 to 4500m3 in 1 month. The leakage alone is about 600m3, which is about 11% of the dam volume in 1 month. That would not be acceptable.
By using these steps, you can see when the dam will be empty, assuming there is no inflow. In practice, the last 0.5m or so of water in a dam is not usable: direct access by livestock will often lead to animals becoming bogged, and mud may clog pumps.
Detailed estimate of water volume
To measure the shape of the base of a square or rectangular dam
Add metre markers on the horizontal string, then:
- Move the dam dipper and measure the depth again until you find the edge of the deepest part of the dam (the edge of the rectangle or square base). Count how many rope loops are suspended over the water.
- Repeat the procedure across the dam until you find the edge of the base on the other side. Again count the number of loops that are suspended over the water. The difference in the number of loops suspended over the water from one side of the base to the other will give you the length of the base of the dam.
- Record the length and depth of the base.
- Repeat steps 1–3 at right angles to your first measurement line. You now have measurements for the base of the dam (length and breadth) and the dam depth.
- Measure the surface dimensions of the water. Pace the length and breadth of the bank at the water surface for rectangular or square dams. Round dams can be measured by pacing the circumference of the bank at water level, then divide this distance by 3.142 (π) to calculate the diameter.
- Insert the figures into the relevant equation below to estimate the current water volume.
Estimate the filled dam water volume
The steps are:
- Measure or pace the sides or circumference of the dam at the elevation of the spillway discharge. This gives you the top measurements when the dam is full.
- Measure the difference in height between the current water surface and the spillway discharge.
- Add this measurement to the current depth to calculate the water depth when the dam is full.
- Insert the new measurements into the appropriate equation below to give you the volume of the dam at full capacity.
Detailed estimate of the partially filled dam water volume
Use the measures of the base and water edge in the equations below.
Volume of a square or rectangular dam
See Figure 3.
Volume (m3) = [A + B + (√ (A x B))] x D ÷ 3
Where:
A = top surface area (m2)
B = base area (m2)
D = depth (m)
For example, for a dam with the water edge 60m long and 40m wide, a 5m depth, and a base 30m long by 10m wide:
Volume = [2400 + 300 + (√ (2400 x 300))] x 5 ÷ 3 = 5914m3 = 5 914 000L
Compare this to the 4 800 000L calculated with the simple formula above.

Volume of a circular dam
See Figure 4.
Volume (m3) = 0.2619 x D x [Td2 + Bd2 + (Td x Bd)]
Where: Td = top diameter (m)
Bd = base diameter (m)
Note: diameter = circumference ÷ 3.142
For example, for a round dam with a top diameter of 48m (circumference of 150m), a 6m depth and a base diameter of 12m:
Volume = 0.2619 x 48 x [482 + 122 + (48 x 12)] = 4752m3 = 4 752 000L
Compare this to the 4 297 000L calculated with the simple formula above.